FB MAFS - 20210722
- XR - XharpRazor Axtra

- Jul 23, 2021
- 2 min read
Updated: Aug 1, 2021
if you are from Facebook, NEWO
if you are here directly, here is the original question:
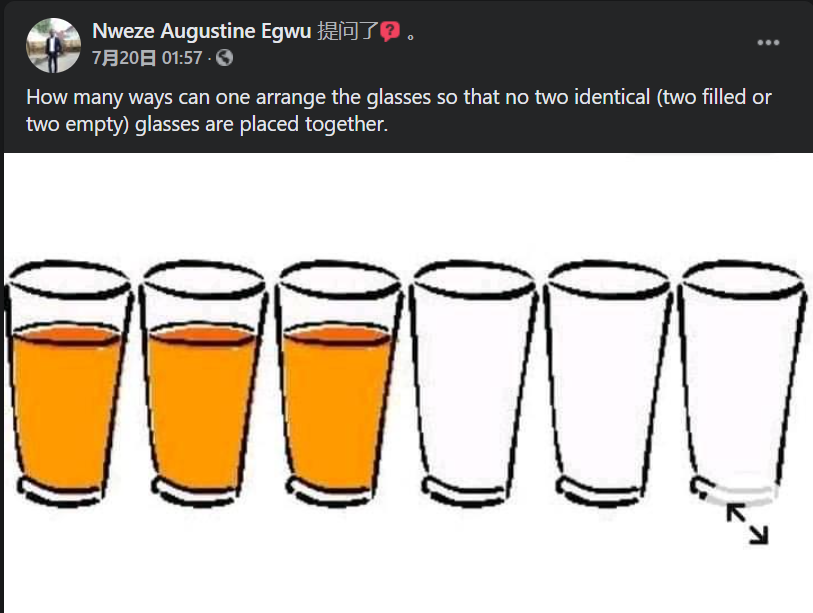
To me, the answer is just "too many ways that I don't even know how to count".
Maybe you think it is just 2 ways :

but what will happen If I do this ?

[1] SETTING UP A FEW RULES
In my case, If I do not follow the rules stated below, My answer would be "Infinite"
1) I have to do this TOPOLOGICALLY in terms of 2 dimensions
since we are dealing with glasses, let's not stack them
(but if you want to play with explosives in a lab with your safety equipment stand by, go ahead),
let's consider we are doing this on a 2D table or any reasonable 2D surface.
2) I will define that "placed together" in the post means that "the glasses are touching each other"
In the post, it didn't define the meaning of "place together",
but according to my common sense, I believe that my definition will be accepted.
If you still don't get it, try to answer this: what does it mean by "together" ? like 10 cm apart? or 1mm apart?
3) I will assume that all glasses are circular and they are identical if looked from above
if we are dealing with weird shapes, this thing is going to be extremely complicated.
[2] WHAT WILL I USE
In this case I will use graphs (not what you see with axis, instead, nodes and edges)
1) each node is a glass,
it could be empty or full, I will use red and blue to indicate that they are different types of glasses, you can say the red ones are empty or full, it is up to you
2) each edge represents that the glasses are touching
[3] WHAT CAN WE KNOW FROM THOSE
from the rules above and from the post, we know that
1) a cycle of 3 or 5 nodes is impossible
since each glasses must contact with a different type of glass
2) a node has a maximum of 3 degrees
from FB, we know that there are only 3 full glasses, 3 empty glasses, a glass only can be surrounded by 3 other type of glasses
[4] HERE IS MY CONCRETE ANSWER
below are my possible methods, each of them is 2D topologically different, I give each configuration a label: I, T, O, X, Q, A, B
if you look very closely, you can notice that Configuration B is extremely special. Still don't know why?

what will happen If I remove edge 1 ? it will become Configuration Q
what about removing edge 3 and 4 ? It will become Configuration I
what about 2 and 1 ? It will become Configuration T
So if you remove enough edges, it can become another answer.
by the way, for Configuration T, "Red is full" and "Red is empty" is different, so configuration T has 2 answers. the same goes for Configuration Q and A
[5] CONCLUSION
So in conclusion, my answer would be "7", or maybe more...
Do you have other answers? Proof me wrong if you can, maybe we can learn something new (OwO)~







Hmm.... but what will happen if I isolate some glasses ? because that also full fill the criteria "no 2 identical glasses put together"...